Strain Energy | Overview and Formula Derivation
- APSEd
- Apr 28, 2021
- 2 min read
What is Strain energy?
Strain Energy is defined as the internal work done to deform a body by the action of externally applied forces.
Let’s say we have an axially loaded bar on which load is applied gradually which increases from 0 to P and due to this load the length of the bar increases by ΔL. The external work done by the applied load will be given as:
External work, W = Average load x Displacement = P/2 x ΔL
Why are we interested in strain energy?
The elastic body has a property - it can store this energy when some external force is applied and this energy can be recovered when this external force is removed - so this energy becomes usable to us.
Strain Energy Formula Derivation
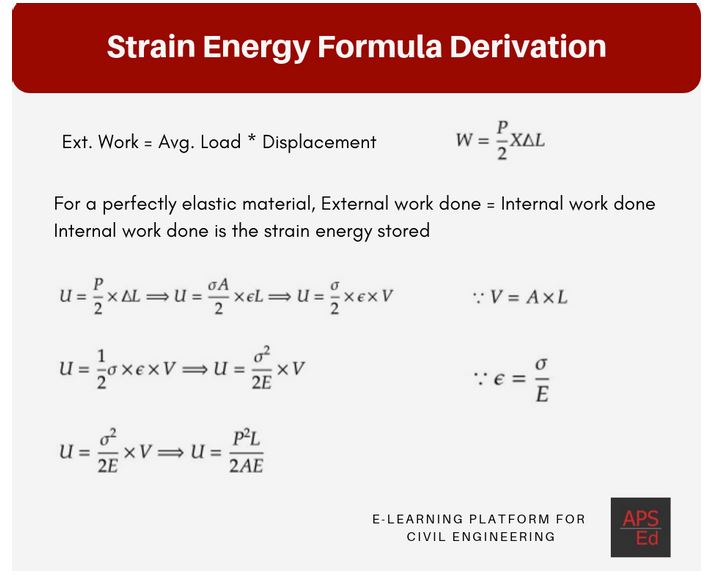
If we assume that there is no energy loss or in other words, if this is a perfectly elastic body then we can say this external work done is equal to internal work done.
For a perfectly elastic material, External work done = Internal work done
According to the definition, internal work done is the strain energy stored.
Strain energy = P/2 x ΔL = 𝜎A/2 x 𝜖 x L = 𝜎/2 × 𝜖 × V = 𝜎/2 × 𝜎/E × V = 𝜎2/2E×V
For unit volume, strain energy U = 𝜎2/2E = P2L/2AE
From the above formula, we can see that strain energy/volume is directly proportional to the square of stress applied and inversely proportional to the modulus of elasticity of a material.
The SI unit of strain energy is Joules/m3.
Now watch this video to visually understand the concept of strain energy and other concepts more clearly.
This is all for now. Let me know by commenting, what all you want next.
Strain energy is the recoverable internal energy stored in elastic materials under load. Learn its formulas and applications through APSEd. Use Mapquest Driving Directions to locate nearby engineering colleges or training centers for further learning.
Really appreciate how clearly this breaks down the concept of strain energy and the derivation process—it makes the theory so much easier to digest. 📐 If you're into applied physics or experimental audio-visual simulations, I stumbled on sprunki mod recently—some of the interactive visual mods there are oddly satisfying and surprisingly educational in their own way.
شيخ روحاني
شيخ روحاني
الشيخ الروحاني
الشيخ الروحاني
رقم شيخ روحاني
شيخ روحاني مضمون
Berlinintim
جلب الحبيب
Really clear explanation — strain energy can be a tough concept to grasp, but this breakdown and formula derivation made it much more approachable. 📐⚙️ Great resource for anyone studying mechanics or structural analysis. On a different note, if you ever need a quick creative break between study sessions, I recently found incredibox— a fun and interactive music game that’s surprisingly relaxing and great for resetting your brain. 🎶😄
New features and material are consistently added to Doodle Baseball through updates, which keeps the game interesting for users who have played it before.